Successive and Finite Difference Method for Gray Scott Model
Keywords:
Partial Differential Equations, Gray-Scott equation, Successive approximation methodAbstract
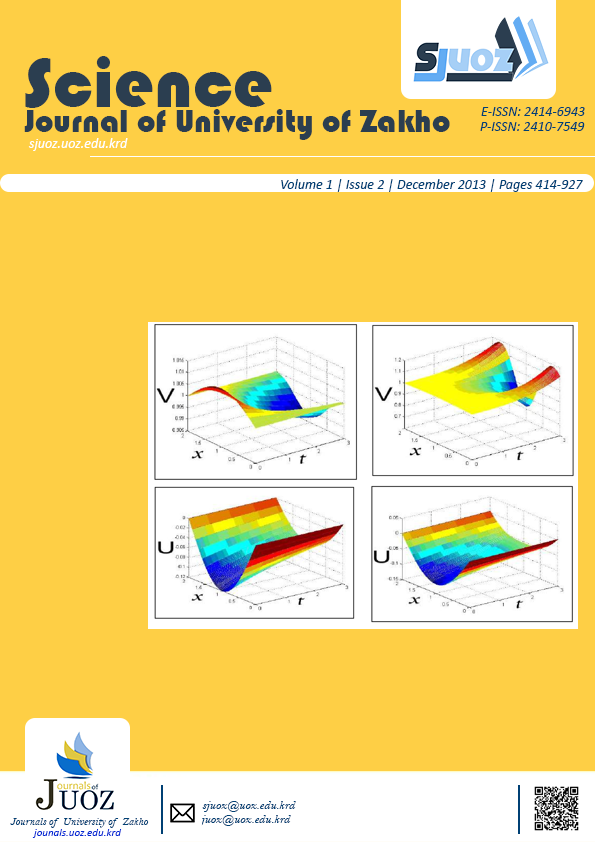
In this paper, Gray-Scott model has been solved numerically for finding an approximate solution by Successive approximation method and Finite difference method. Example showed that Successive approximation method is much faster and effective for this kind of problems than Finite difference method.
References
Brauer, F., & Nohel, J. A. (1973). Ordinary Differential Equations: a First Course (2nd ed.). INC. London: W. A. Benjamin.
Chakravarti, S., Marek, M., & Ray, W. H. (1995). Reaction-diffusion system with Brusselator kinetics: Control of a quasiperiodic route to chaos. Physical Review E, 52(3), 2407-2423.
Coddington, E. A. (1961). An introduction to ordinary differential equations. Englewood Cliffs, N.J.,: Prentice-Hall.
Cross, M. C., & Hohenberg, P. C. (1993). Pattern formation outside of equilibrium. Reviews of Modern Physics, 65(3), 851-1112.
Guymon, G. L. (1970). A finite element solution of the one-dimensional diffusion-convection equation. Water Resources Research, 6(1), 204-210.
Jerri, A. J. (1985). Introduction To Integral Equations With Applications. New York & Basel: Marcel Dekker, Inc.
Lawrence, C. E. (2010). Partial Differential Equations (2nd ed. Vol. 19): American Mathematical Society.
Leon, L., & George, F. P. (1982). Numerical Solution of Partial Differential Equations in Science and Engineering (1st ed.): Wiley-Interscience.
Logan, J. D. (1987). Applied Mathematics: Wiley-Blackwell
Mathews, J. H. (2004). Numerical Methods Using Matlab: Pearson.
Mingjing, S., Yuanshun, T., & Lansun, C. (2008). Dynamical behaviors of the brusselator system with impulsive input. Journal of Mathematical Chemistry, 44(3), 637-649.
Murray, J. D. (2007). Mathematical Biology: I. An Introduction (3rd ed.). New York: Springer.
Nicolis, G., & Prigogine, I. (1977). Self-organization in nonequilibrium systems : from dissipative structures to order through fluctuations. New York: Wiley.
Otto, S. R., & Denier, J. P. (2005). An introduction to programming and numerical methods in MATLAB. London: Springer.
Saeed, R. K. (2006). Methods for solving system of linear Volterra and integro-differential equations. Salahaddin University, Erbil, Iraq.
Shanthakumar, M. (1987). Computer Based Numerical Analysis: Khanna.
Temam, R. (1997). Infinite-dimensional dynamical systems in mechanics and physics (2nd ed.). New York: Springer.
Chakravarti, S., Marek, M., & Ray, W. H. (1995). Reaction-diffusion system with Brusselator kinetics: Control of a quasiperiodic route to chaos. Physical Review E, 52(3), 2407-2423.
Coddington, E. A. (1961). An introduction to ordinary differential equations. Englewood Cliffs, N.J.,: Prentice-Hall.
Cross, M. C., & Hohenberg, P. C. (1993). Pattern formation outside of equilibrium. Reviews of Modern Physics, 65(3), 851-1112.
Guymon, G. L. (1970). A finite element solution of the one-dimensional diffusion-convection equation. Water Resources Research, 6(1), 204-210.
Jerri, A. J. (1985). Introduction To Integral Equations With Applications. New York & Basel: Marcel Dekker, Inc.
Lawrence, C. E. (2010). Partial Differential Equations (2nd ed. Vol. 19): American Mathematical Society.
Leon, L., & George, F. P. (1982). Numerical Solution of Partial Differential Equations in Science and Engineering (1st ed.): Wiley-Interscience.
Logan, J. D. (1987). Applied Mathematics: Wiley-Blackwell
Mathews, J. H. (2004). Numerical Methods Using Matlab: Pearson.
Mingjing, S., Yuanshun, T., & Lansun, C. (2008). Dynamical behaviors of the brusselator system with impulsive input. Journal of Mathematical Chemistry, 44(3), 637-649.
Murray, J. D. (2007). Mathematical Biology: I. An Introduction (3rd ed.). New York: Springer.
Nicolis, G., & Prigogine, I. (1977). Self-organization in nonequilibrium systems : from dissipative structures to order through fluctuations. New York: Wiley.
Otto, S. R., & Denier, J. P. (2005). An introduction to programming and numerical methods in MATLAB. London: Springer.
Saeed, R. K. (2006). Methods for solving system of linear Volterra and integro-differential equations. Salahaddin University, Erbil, Iraq.
Shanthakumar, M. (1987). Computer Based Numerical Analysis: Khanna.
Temam, R. (1997). Infinite-dimensional dynamical systems in mechanics and physics (2nd ed.). New York: Springer.
Downloads
Published
2013-09-30
How to Cite
Manaa, S. A., & Rasheed, J. (2013). Successive and Finite Difference Method for Gray Scott Model. Science Journal of University of Zakho, 1(2), 862–873. Retrieved from https://sjuoz.uoz.edu.krd/index.php/sjuoz/article/view/433
Issue
Section
Science Journal of University of Zakho
License
Authors who publish with this journal agree to the following terms:
- Authors retain copyright and grant the journal right of first publication with the work simultaneously licensed under a Creative Commons Attribution License [CC BY-NC-SA 4.0] that allows others to share the work with an acknowledgment of the work's authorship and initial publication in this journal.
- Authors are able to enter into separate, additional contractual arrangements for the non-exclusive distribution of the journal's published version of the work, with an acknowledgment of its initial publication in this journal.
- Authors are permitted and encouraged to post their work online.
